Both those "anomalies" were at the level of 2-sigma or so -which means the data had a probability in the 1-5% range of being observed like that if the Standard Model was correct. And now, it is CMS turn: there is a 2.5 sigma excess of lepton-flavour violating decays of Higgs bosons to get engrossed with, as found by a new CMS analysis.
I feel I need to apologize for firing up two "exciting" posts in the matter of three days here, but the fact is, particle physicists tend to accumulate their results in two distinct occasions six months apart: summer conferences and winter conferences. This allows them paid vacations to fancy skiing resorts or other appealing travel locations (for those who like to have a life), or to blend in with hundreds of colleagues in huge conferences and sit at scores of talks (for those who are serious about their job). So it happens that large collaborations present many interesting results (and many less interesting ones) all at once, where they can have the smallest possible impact on the media circus. I never got around to understanding why that is supposed to be a good thing, but that is what happens and so let's ride it.
Lepton-Flavour-What ?
Today's result comes from the search for an exotic possibility: can the Higgs boson decay to a tau-lepton and a lighter lepton ? Of course not. Not in the Standard Model, at least: it would imply that the Higgs boson possesses the alchemic power of transmuting matter, even more so than can the W boson. Hmm I am being cryptic, so let me explain.
While matter particles do decay -most of the times at blitzing speed- into lighter ones, they have been observed to preserve some characteristics: mother passes to daughter some property which does not get lost. So, for instance, when a muon decays to electron, electron-antineutrino, and muon neutrino, the "muon-ness" is passed from the muon to the muon neutrino. Or when a neutron decays to a proton, an electron, and an electron antineutrino, the "baryonic number" of the neutron is transferred to the proton. Matter changes, but can't be destroyed. And even when it changes, it does so by following that inheritance rule of "good quantum numbers".
The particle that transmutes matter is the W boson: by coupling to a W the muon-ness is transferred to the muon neutrino. And by coupling to a W, the down-quark in the neutron becomes a up-quark, still holding on its 1/3rd of baryonic number despite changing nature. Indeed, we have never observed baryonic-number violations or leptonic-number violations so far: the conservation of these properties -their passing on to daughters in decays- has never been falsified.
Take the Higgs boson now. What does it have to do with all of the above ? The Higgs does not perform any decay trick like the W: it cannot, by "coupling" to a particle, change the nature of the latter. If it attaches to a top quark, the latter remain a top quark; if it is emitted from a muon, the muon remains such. All of this is so intrinsically wired in the equations that govern the Standard Model that thinking otherwise is anathema. Inventing the Higgs boson to solve the puzzle of electroweak symmetry breaking was a mindboggling feat -I would rate it among the 10 greatest accomplishments of the human mind; but inventing it to solve a puzzle and then finding out that things work out that way by being much more complex would be a bit disturbing.
So we rest assured that the Higgs does not, in fact, decay to a tau lepton and a muon, as this would really make no sense. Or maybe it would ? In fact, our theorist friends like to speculate, and some of them explain to us that it would indeed make sense. There are models that include lepton-flavour-violating Higgs decays without breaking up any of the niceties of the whole construction; models with more Higgs bosons, or models with composite Higgs bosons... I believe I do not need to enter in the details. So let us look at the data instead.
The New CMS Analysis
First of all, one needs to remember that a limit on lepton-flavour-violating Higgs decays was set at <13% by ATLAS in their H->tau tau search. CMS considered the direct reconstruction of Higgs decays into a tau lepton and a muon, by finding taus either as narrow jets of light hadrons (something that taus do quite frequently) or as a single electron. Why not in tau -> μ decays ? Because that would give a dimuon final state, which is much more background-ridden.
It transpires that the two-tau background is one major obstacle in this search: tau-lepton pairs can be produced by a Z boson, and one of them may decay to a muon, while the other may give rise to the tagging signature required by the analysis (a narrow jet or an isolated electron). However, the muon from a lepton-flavour-violating decay is much more energetic than the muon emitted by tau decay, as tau decay must share the tau energy (say, half the Z mass if it comes from a Z boson, or half the Higgs mass if it comes from a H) into three decaying objects - the muon, the muon antineutrino, and the tau neutrino. For remember: the tau does not violate lepton flavor in its decay, so "tauness" is transferred to the tau neutrino. It is the same thing that we discussed above with the muon decay.
CMS studies all backgrounds with care and produces a detailed prediction for each. One typical trick is to look for tau leptons and muons which have the same electric charge: that just cannot be the result of a Higgs boson decay -it would be asking for too much violation in a single decay!!- so by comparing data and simulations in such a control sample devoid of signal it is possible to verify that backgrounds like fake muons (which know nothing about the charge of the tau, e.g.) are under control.
Of course, the variable which is looked at is the invariant mass of the tau and the muon -doh... It would obviously peak at 125 GeV in case of a Higgs decay, while all backgrounds either peak elsewhere (if the tau plus muon come from a Z boson decay, where the muon comes from a tau decay) or not peak at all (like, for instance, misidentified leptons).
In the end the mass spectrum is the one shown below. Or rather, the mass spectra: the Higgs selection produces several possible signal-enriched categories, including Higgs candidates produced together with two forward hadronic jets (the so-called "vector-boson-fusion" production process yields two such tagging jets). By dividing the data into 0, 1, and 2-jet categories and considering separately the "narrow jet" and the "electron" tau signatures, six separate histograms are produced. Note as the various backgrounds contribute differently in the six subsets of the data.

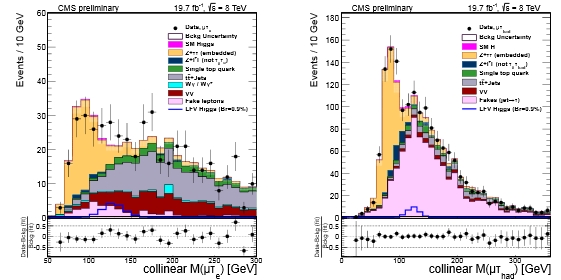
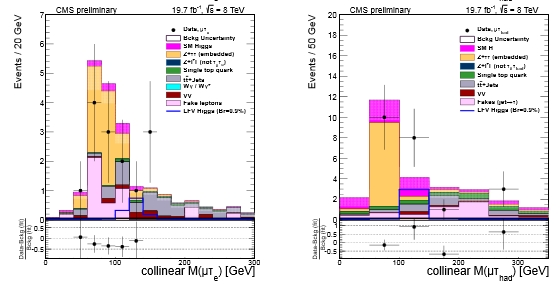
(In all the six graphs above, the top panel shows the mass histogram, and the bottom panel shows the residuals between data and sum of backgrounds divided by the background).
If one compares the data (black points) to the sum of backgrounds (coloured histograms) one observes that there is a mild excess around 125 GeV in both 0-jet categories (the two top panels) as well as some less significant excess in the 1-jet mu-tau_e and 2-jet mu-tau_had categories (center left, bottom right respectively). Note that in the graphs the possible lepton-flavour-violation Higgs signal has been overlaid as an empty blue histogram at the bottom; the branching fraction hypothesized for the histogram is a mere 0.9% - 15 times smaller than the ATLAS limit.
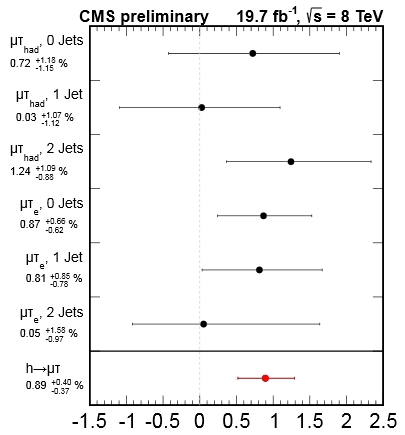 Using the data and the complex combined likelihood fitting procedure that has discovered the Higgs boson two years ago, CMS produces an estimate of the branching fraction in each of the categories and then as a combination of all. It is shown in the graph on the right.
Using the data and the complex combined likelihood fitting procedure that has discovered the Higgs boson two years ago, CMS produces an estimate of the branching fraction in each of the categories and then as a combination of all. It is shown in the graph on the right.As you can see, while error bars are too large to speculate too much, one can see that there is a consistent picture of non-zero branching fraction. Of course the Standard Model (which predicts the branching fraction to be exactly zero) is by no means ruled out - the effect is at the 2.5 sigma level, which means that there is a chance of 0.7% of observing data at least as discrepant in this search.
The significance of the measurement, in my humble opinion, is not so much in the slight excess from predictions, as in the much tighter limit that CMS could set on lepton-flavour-violating decays of the Higgs: from the 13% limit of ATLAS, we have gone down by one order of magnitude: CMS excludes LFV decays with a branching fraction exceeding 1.57%, at 95% confidence level. And of course, at this point it will be very interesting to see what ATLAS can do by using the same technology: if they, too, saw a 3-sigmaish excess, there would really be room to get excited again!




Comments