A one-picobarn cross section means that ZZ production is a quite rare process at a hadron collider: only one collision in a hundred billions produces that final state. Furthermore, Z bosons prefer to decay into jet pairs than into the easier-to-spot charged leptons which typically make out the golden "Z samples": electron-positron pairs, or muon pairs. The possible decay modes of the Z boson involve quarks 70% of the time, neutrino pairs 20% of the time, and electrons, muons, and taus 3.3% of the times each. Of course, the jet final state is quite tough to put in evidence, because of the huge rate of background events from quantum chromodynamics; taus are also disfavoured for much the same reason.
If you are going to look for a ZZ pair, you may further be tempted to avoid using the 4-charged-lepton final state, which is extremely clean but quite rare! Let us see just how rare that is.
Imagine that to spot ZZ pairs you restrict yourself to counting events with four muons, or four electrons, or two muons and two electrons: these channels have relative probabilities of 0.033^2, 0.033^2, and 2*0.033^2 with respect to all ZZ pairs produced at the Tevatron. These numbers are 0.0011, 0.0011, and 0.0022 respectively, or if you add them up they make 0.0044: so you are looking for a one-in-hundred-billion final state, and you discard 99.5% of it ? There must be a better way.
Indeed there is. If you require that one of the Z decayed to electron-positron or muon pairs, while the other decayed to neutrinos, the resulting final state is not as rare as the 4-charged-lepton one: the probability that a ZZ pair decays that way is 2x0.066x0.20=0.0264 (the first factor of 2 comes from allowing either Z boson to give electrons or muons). This, while small, is six times larger than the 4-lepton mode. Let us then compute how many such events are produced in a dataset of 5.9 inverse femtobarns of collisions -the one used by CDF in the search.
The number of events is always given by the cross section times integrated luminosity: so we get N=5.9/fb x 1.2 /pb x 0.0264, where we have factored in the total branching ratio to leptons and neutrinos computed above. Since one inverse femtobarn is a thousand inverse picobarns, we figure that N turns out to be about 190. A sizable signal! However, that number is not realistic for two reasons. The first is that not all those events will be collected, due to the limited acceptance of the CDF detector to charged leptons, and to the possibility that neutrinos produce a insignificant amount of missing energy (for instance, by being almost back-to-back in the transverse plane, or by escaping along the beam line). The second is that a dilepton plus missing energy signal is not as clean as the 4-lepton final state, and a tight additional selection is required to extract it from backgrounds.
Backgrounds producing two leptons and significant missing energy (the tell-tale sign that energetic neutrinos have escaped the detector unseen) come from many different sources. The most important are due to single Z production and to WW pairs. Z production may produce two charged leptons, and some additional jet activity may produce the missing energy signature if the calorimeter response to the jets fluctuates high or low. WW pairs instead produce naturally the dilepton plus missing energy signature; the two leptons, however, are often of different kinds -while a Z only decays to ee or mu-mu pairs- and further, their combined invariant mass does not always give a value in the ballpark of 91 GeV, as is in the case of dileptons from Z decay.
There are many additional handles to separate ZZ signal events from the quoted backgrounds and from additional ones (such as top-antitop production, for instance). The analysis utilizes a NeuroBayes(TM) neural network to discriminate them. The network receives as input the observed event kinematics, and in particular opening angles of the leptons, missing energy and its significance, and transverse momentum of the dilepton pair. While the choice of these variables is very conservative and sound -they are all well modeled by simulations-, one might object that they select ZZ pairs due to standard model processes, so new physics producing ZZ pairs might be discarded by such a selection. In this sense, the search is really a check of the Standard Model rather than a search for new physics.
The output of the neural network is shown in the figure below. As you can see, there is a clear signal from ZZ-like events at the highest values of the NN discriminant, on the right (in red). Other things to note are that the data follows very neatly the background expectation, and that the highest signal-to-noise bins contain a fraction of signal amounting to roughly 30%. This is a nice result, showing that the neutrino signature is worth pursuing when the decay of a heavy object is implied.
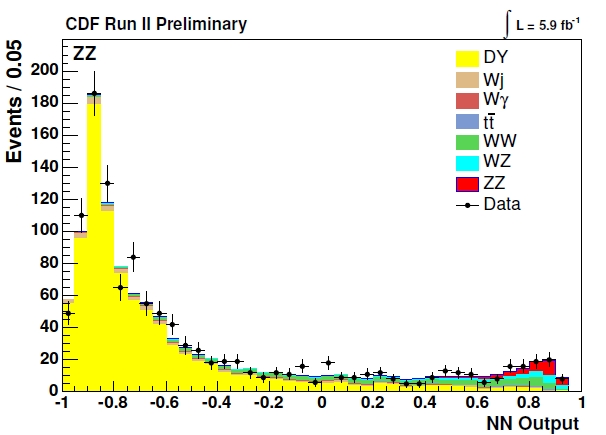
In summary, ZZ events are observed cleanly in the dilepton plus missing energy final state in a dataset of almost 6 inverse femtobarns of proton-antiproton collisions. The cross section agrees with the Standard Model - but that does not imply much for new physics in my humble opinion. Finally, I congratulate my colleagues in Padova who contributed to this new interesting result from CDF. The analysis details can be found in a public note here.




Comments