Be warned: this article deals primarily with shark attacks, the lottery, beer, and how to get a date using math. Is it a good decision to keep reading? Unfortunately, the answer is "you need to keep reading to find out."
Sound irrational? Good—your massively irrational mind should have no problem with it, then.
Consider this: every year when the Discovery Channel broadcasts "Shark Week" visits to Florida beaches decline. Presumably, the network's programming makes the waters no less safe (assuming sharks are not, in fact, empowered by cable television). However, after watching a week of kicking legs seen from below, the idea of shark attack is refreshed in our minds and we choose not to offer ourselves as bait.
This phenomenon is known as an availability heuristic — a heuristic being a rule-of-thumb. Our rationality is subverted by easily available sensationalist images.
On the sunnier side of the availability heuristic is the lottery. Should you invest $5 a day or use it to buy lottery tickets?
Math makes the decision obvious. Suppose you invest five bucks every day at the not-unheard-of rate of 10%. It will take you almost exactly 40 years to accumulate $1 million. To earn this same $1m playing Powerball, you would have to match five balls and the Powerball at odds of one-in-146,107,962. Spending this same $5 a day to buy 73,000 lottery tickets over 40 years gives you about a 1/2000 chance of winning the jackpot. Granted the Powerball jackpot is likely over a million (even after taxes, payouts over time, and splitting with other winners), but there’s no way it’s 2,000-million and thus investing the money wins no matter how you slice it. (Yes, yes—this is oversimplified, but while adding complexity clouds the result, it doesn't change it.)
However, the available image of immediate wealth subverts this rationality; when we picture the payout, our minds go wonk.
Alphabetically, the availability heuristic is only the first in a long line of psychological mechanisms that lead us into bad decisions. Imagine—if you will—beer. See, wasn't that nice? Now imagine sitting in front of the taps at your local watering hole (nice again, eh?). Which beer do you like best? If you are like most human beings, the answer is "the most expensive one." A number of studies have shown that by switching price tags, you can switch preferences (for obvious reasons, this is a favorite experiment among university psychology students). This works with other beverages as well—just ask Starbucks.
And what about the power of suggestion?
Imagine I handed you a cup of hot (Starbucks) coffee and then asked your opinion about a person whom you had recently met; now suppose I instead handed you a cup of ice-cold soda. Experiments show that your opinion of this person would be different because you have been primed to feel warmth or coldness.
Add to the list...
* framing (how you present data is as important as the data itself)
* impact bias (overestimation of possible outcomes),
* confirmation bias (recognizing only data that supports your hypothesis)
* loss aversion (we stand to gain more than we would lose, but our fear of loss prevents us)
* selective perception (seeing what you want to see),and
* rosy retrospection (integral to the repeated experience of family Christmas)
...and you seem doomed to blunder through life led by your brain's clumsy irrationality.
Is there any hope for the human race? In the example of the lottery, mathematics offered incontrovertible rationality. Might we be able to apply mathematics to other situations as well?
A rudimentary attempt at this is the list of plusses and minuses, in which one lists the positive aspects of a decision on one side of a chart and the negative aspects on the other, and then weighs these against each other as if on a scale (the heavier side wins).
To add a layer of mathematics, if one factor on the list is more important than the others, we might multiply it by two. If it is very important, we could even square or cube it.
Suppose you were sitting in the aforementioned bar, drinking the aforementioned beer (perhaps while holding the aforementioned Powerball ticket and worrying about the aforementioned shark), while sneaking peeks at a beautiful woman sitting at the bar. What do you think would influence your chance of success with this woman?
It will certainly help if you are attractive—especially in comparison to her (you might say your chances increase in direct proportion to your looks/her looks); it will also help if you are a witty conversationalist and willing to pursue the interaction aggressively, and hurt your chances drastically if she already has a boyfriend (esp. a large one).
Putting this into an equation, we could come up with the following (W=Witty, G=Aggressive, Ay=Your Attractiveness, AH=Her Attractiveness, R=Her "Amount" of Current Relationship; all variables from 1-10 with 10 being high):
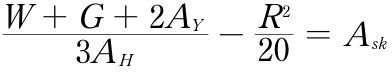
You would, of course, have to evaluate the results on some type of scale, like the one here:
* If ASK is less than zero you should lower your standards
* If ASK is between zero and 1, you have exactly a snowball's chance in hell with her
* If ASK is between 1 and 10, game on!
* If ASK is greater than 10, consider her more attractive friend instead
(Can somebody hit me with a widget here?)
Is this science? Is this math? Is this valid? Completely valid or valid in concept? Are we naught but zombific, perambulatory computers translating input into output with predestined results?
To find out the answer (and to watch geeks armed with hidden cameras and calculators apply Geek Logik formulas at the singles bar, followed by a segment of me trying—and failing horribly—to affect womens' shoe-buying decisions in an upscale NYC boutique), watch the BBC Documentary "Foolproof Equations for a Perfect Life" on the Science Channel on Sunday, June 15 2008 at 9:00pm EST.




Comments