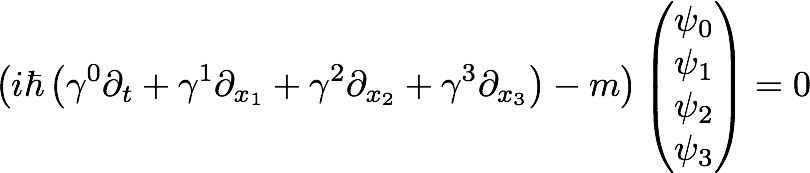
It is a zoo of gamma matrices that I have trouble keeping track of. There are a variety of identities one needs to prove. There are 4 gamma matrices, but don't forget about the fifth one. The fifth one doesn't belong in the Clifford algebra, but is useful for studying helicity. But to have a complete set, meaning that any constant 4x4 matrix can be represented by a linear combination of these matrices, then that complete set has 16 Dirac matrices.

I spent more than an hour writing that last paragraph, reading and rereading descriptions of the gamma/Dirac matrices, trying not to get confused about all the relationships. I have done that in the past too, but it still feels like too much math for me to keep track of.

So I am going back to the quaternions, and more importantly, physics.

To describe the atom smashing in bowels of the LHC, we need all possible histories.

That is the physics.

Start simple:

This is the couch potato transformation - nothing happens.  That I understand (and the same thing does happen with gamma matrices).

Readers may quickly guess that I could multiply on the left by one of 4 basis vectors, and do the same on the right, for a total of 16 possibilities. Would that magically make up the complete set of 16 Dirac matrices that can represent any constant 4x4 matrix?

This was not my idea. As the owner of quaternions.com, I get papers sent to me as an "internet authority" on the subject. The paper was titled: "Dirac matrices using quaternions" by J. López-Bonilla and L. Rosales-Roldán. In hindsight, I should have question the paper based on the title alone. The Dirac matrices are the Clifford algebra CL(1,3) while quaternions are CL(0, 2). The two are never going to be the same.

One of the many subjects I have not studied in math is all the meanings of "the same", or more technically, morphisms. I have heard of the variety of them (iso, endo, auto, …), but have trouble getting them right. With considerable aid from David Halliday, I did figure out how exactly to go from the map suggested in the paper to the gamma matrices. It requires 5 extra factors of i.

Like the Klein-Gordon equation, the quaternions get the signs and factors of i wrong. We know the conventional Klein-Gordon equation and gamma matrices work together to do useful calculations. It is merely a belief on my part based on the ability to construct the map above that the quaternion not-KG and quaternion-triple-permutation-product-that's-not-gamma could be used in relativistic quantum field theory calculations. I work in too much isolation to work with someone on a demo calculation to give it a thumbs up or down.

From now on, I will assume that there is a way to use quaternions, odd sign conventions and all, to do useful calculations.

Let's work through the four "quaternion gamma's" to see what happens. First off is gamma zero:

My first response to this is simple: this is crazy complicated.  Well, it should be to keep supercomputers busy for months on end. But that was an over-reaction.  The source equations for the Maxwell equations in the Lorenz gauge in this pedantic format looks like so:

This of course is second order instead of first order, yet there is a clean organization of terms. No wonder light is easier to deal with than electrons.

There are 3 other gammas.  Is anything important accomplished by doing them?  No, the story is the same, only the signs and locations of terms varies (each of the 16 terms has to be somewhere, just somewhere different).  Yet I will do the work because people learn through repetition, so here I go again with gamma 1:

Things stuck out when I did this calculation. When filling in the 0-3's at the end, I used the same LaTeX code as gamma 0, but traded 3's for 0's and 1's for 2's.  The final line felt particularly odd, but that is the way the partial derivatives get all scrambled up.

Note: this really is a Sudoku puzzle. I checked for one partial of each of the four times, one phi in each row or column.  Doing that check in the first draft indicated an error (two psi 0's in one line).

On to gamma 2!

This was similar to the last one, only some signs were changed.  Anything with a partial of zero or two flipped signs.  One more to go…

This time the signs stayed in the same places, but there was a swap of 0 for 1 and 2 for 3.

Nature has the capacity to keep track of this level of detailed variation, although I do not.  I did notice that all four equations had one term with three minus signs, one with three positive signs,  and two with one minus.

Scrambled Eqs

Light is comparatively simple: the same operator acting separately on one part of the potential at a time.  The equations for matter look like they are the light equations that are systematically scrambled.  I like that result because we know photons interact in many ways with matter.

A core difference between bosons and fermions is that for bosons, it takes the expected 2 pi rotation to get back to the starting place. Fermions take twice as much rotation. Sometimes this 2x requirement is presented as an impossible thing to understand, part of the magic of quantum mechanics. Actually, it happens for any cup you put in your hand. Sure, if you sit on a bar stool and spin around, it only takes 2 pi rotations.  You and the cup together are free.  But try to do the same where you don't move but the cup does.  After 2 pi rotations, your hand and arm are not back where they started.  That is due to the complications your shoulders create.  When I look at the equations for Maxwell in the Lorenz gauge, they look like they are free to return after 2 pi rotations. The "quaternion Dirac" equations look like the signs will get all tangled up.  Go around twice and two sign changes do make a positive.

Caveats, Caveats

I admitted I cannot do a calculation with any of the equations presented. If you choose to look at this blog as a silly game of quaternion gamesmanship, I can accept that position. When working exclusively in four dimensions as happens with the Klein-Gordon and Dirac equations, I could not justify avoiding a division algebra as it is manifestly a more powerful math tool. Granted, history is so on the side of tensors. If you were so motivated, I do think all references to quaternions could be deleted from this post, but a core message - the Maxwell equations in the Lorenz gauge is neatly separated, while the Dirac equation mixes things up - remains.

Why do only 4 of the 16 gamma matrices (mine or the standard set) get used?  My guess would be that a different set of four could be used, but that is only a speculation.  There may well be redundancy with the full 16.

The subject of helicity was not brought up.  That might be fun to explore in a different blog.  That process uses one plus or minus the non-CL(1,3) gamma 5.  That is a big topic, this blog is long enough.  I hope you see new relationships between the equations for light and matter.