This is just a fun question someone asked on Quora. It leads into a few interesting topics. First the ISS just isn't heavy enough for a satellite to enter into a true gravitational orbit around it. But, in theory at least, you can put a satellite into a temporary  orbit that looks from the point of view of the ISS as if it is in orbit around it.

For a while. It's not kept in place by any gravitational attraction to the ISS, so it is sort of an illusory orbit you could say in a way - but perhaps it is interesting.

ORBITING THE ISS BY COINCIDENCE

The trick is to put it into an elliptical orbit that has exactly the same period as the ISS, so same major axis. Then make it so that it goes, say, 50 kilometers above it at one point in its orbit, and 50 kilometers below it when it is the opposite side of the Earth. And then get them exactly in the right phase relative to each other.

Then, because it travels around the Earth more slowly than the ISS when further from the Earth, and more quickly than the ISS when closer to the Earth (by Kepler's "equal areas in equal times"), it will seem to follow a retrograde orbit around the ISS when seen from the ISS.

There is no gravitational attraction involved here. It is just a coincidence, and it will continue to appear to orbit the ISS in a retrograde direction, from the point of view of the ISS, for as long as you can keep the two orbits with exactly the same period. But the periods can't be exactly the same, apart from anything else, there is a significant amount of drag and it's almost impossible for this to be the same for both satellites. 

So eventually it will drift out of sync, unless of course you do continual small corrections to keep it in its "orbit" - and there will be a risk of it hitting the ISS. So in practice nobody would put a satellite into such an orbit, at least, not permanently.

As for a true gravitational orbit, the "orbit" would be very slow because of its slight gravity, and soon disrupted by tidal effects from the gravity of the Earth. You can work it out here:  Orbital period of a planet. Put the mass of the ISS as 370,131 kg and orbit at a distance of 50 meters and the orbit of a small satellite, of 1 kg, is a bit over 5 days. 

But the orbit of the ISS is just 90 minutes.

You can work out the sphere of influence of the ISS, for that mass, using this online Hill Sphere calculator, put in semimajor axis of the Earth as 6738 km and you get a "hill sphere" for the ISS of a bit short of 185 cm.

So - no way that anything can orbit it for long at a distance of meters based on its gravitational attraction. Orbits like that would work in free space, but the nearby Earth with its tidal effects will disrupt them.

SIMILAR ORBITS FOR THE MOON

However orbits a bit like this are useful for the Moon. They are called "lunar retrograde orbits". In this case, the lunar gravity also helps to keep them in sync. They are the result of three body simulations so are not perfect keplerian orbits, but rather rosette like orbits.

Here is a simulation of a Distant Lunar Retrograde Orbit.


If the ISS did have enough gravity to have a temporary satellite, it would then be a three body problem, so it would probably go into a rosette type orbit like this.

They may not be permanent orbits of the Moon, but they remain stable for a century or more. One idea for NASA's asteroid retrieval project is to return it to a lunar retrograde orbit.


Here the Distant Retrograde Orbit (DRO) is shown orbiting the Moon just outside the lunar L1 and L2 positions. It can also orbit inside them, or it could even go into very large orbits that go nearly down to the surface of the Earth if you so wished. The orbit shown can be stable for hundreds of years - see Research into NASA's Asteroid Redirect Mission. 

NOT LIMITED BY ITS HILL SPHERE

Usually satellites of a moon or planet can only orbit inside a limited region called its "Hill Sphere". But these orbits are not limited by the hill sphere of the Moon, though they are also not long term stable. 

You can have a lunar retrograde orbit even going all the way down to just above LEO, and from the point of view of the Moon it is similar to a satellite orbiting the Moon, though from the point of view of the Earth it is a satellite in a 28 day extremely elliptical orbit around the Earth that sometimes passes in front of the Moon and sometimes passes behind it (though following a more complex trajectory because of the three body interactions).

So, it works for the Moon, at least for a century or so.

But you can't keep a satellite in an orbit like that around the ISS for long, because it hasn't got anything like the gravity of the Moon. It also orbits close to the Earth where atmospheric drag is significant - and the ISS keeps doing boosts to keep in a high enough orbit. There is no way a satellite of the ISS could follow when it does that.

RETROGRADE ORBITS AROUND MOONS OF JUPITER, SATURN ETC

For similar reasons, retrograde orbits around moons are more stable than prograde orbits (orbits in the same direction as the orbit of the moon around a planet). If you want to send a spacecraft to orbit Europa, or Ganymede or Enceladus, you'll probably use a retrograde rather than a prograde orbit of the moon (though of course still a prograde orbit of Jupiter or Saturn).

This shows an idea for a distant retrograde orbit around Europa in inertial frame (left) and rotating frame centered on Europa (right).

And the same is true for planets around the sun.

The natural moons orbit in the same direction as the planet,  so are always prograde. 

But many of the captured moons, are in retrograde orbits, as these are easier to get into and more stable, especially if you are far from the planet.

HAS ANYONE PUT A SPACECRAFT INTO A TEMPORARY "ILLUSORY ORBIT" AROUND A SPACE STATION?

For multiple reasons all of this is impossible for the ISS. Except for a short time, a few orbits, as a coincidence.

If someone wanted to, they could put a Soyuz, say, into such an "orbit" around the ISS for at least a few orbits of the ISS (looks like an orbit of the ISS as seen from the ISS that is, just a slightly elliptical orbit of the Earth as seen from Earth) though I don't know if this is ever done, if there is any reason to do it, rather than just approach the space station directly.

Do say in the comments if you know if anyone has put a Soyuz or other spacecraft into a temporary retrograde "orbit" around the ISS (or other space station such as MIR) in this way. Or any other comments or corrections of course :).

GRAIN OF SAND ORBITING A BOWLING BALL IN GEO

By the way, you could also put a tiny satellite into orbit around a very small very dense satellite. 

Let's try a bowling ball and see if you could get a grain of sand to orbit it.

Using this online Hill Sphere calculator, putting the mass of the bowling ball as 7.26 kg, and then at geostationary orbit around the Earth, its hill sphere is a bit over 31 cm. Or a little over 62 cm diameter.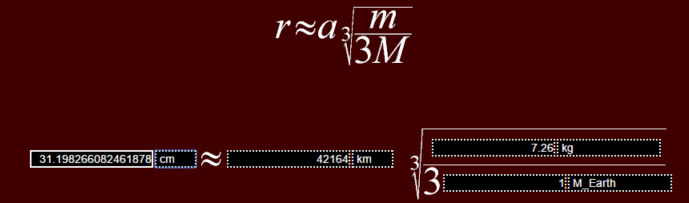 

Since a bowling ball is at most 21 cm in diameter, that suggests that a grain of dust carefully placed into orbit around a bowling ball in geostationary orbit around the Earth  (at a height of 35,000 km above the Earth) would be well within its Hill Sphere so might continue to orbit it for at least a while until disturbed by solar radiation or some such.

Using this online orbital calculator: Orbital period of a planet, and supposing the grain of sand has a mass of a milligram, the orbital period of our grain of sand is  2 hours, 41.86 minutes.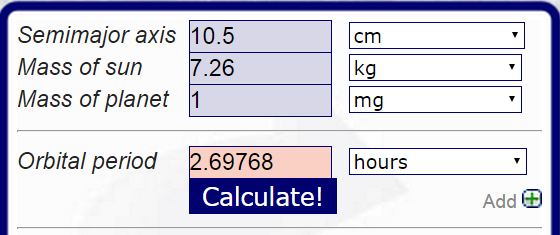 

It doesn't need to be quite as small as that. 

It has a similar orbital period if its mass is a gram. If its mass is a kilogram, orbital period 2 hours and 31.75 minutes.

Actually, there is a rather nice short cut here. In the formula for the orbital period of a satellite, two body problem, if you have a satellite orbiting just above the surface of a planet, so a is the radius of the planet - then the formula for its orbit simplifies to


an expression with no mention of the radius - where ρ is the mean density in kg / m3. See Orbital period as a function of central body's density

In other words, for LEO the orbital period depends only on the mean density of the body, not on its size. Any planets or moons with the same density will have the same periods for low orbits.

We can take this right down to miniature levels. If you can replace your bowling ball by a sphere with the same density as the Earth, then the grain of sand, or your one gram weight even, would orbit with the same orbital period as the ISS orbits around the Earth.

The density of the Earth is 5.5 grams / cm3. So - that's in between the density of the densest rocks and the density of zinc (also much more dense than aluminium).  It's about the density of high end lead glass, so make your sphere out of high end lead glass, and the orbital period of your small grain would be the same as the ISS.

More generally, by similar argument, you could make a miniature version of the solar system in free space. If you could replace all the bodies in our solar system, including the sun, by smaller versions of themselves with the same density, and if you then reduce the distances between the planets and moons in the same proportion - then the orbital periods of all the planets and moons would remain unchanged :).

WHAT ABOUT ASTRONAUTS INSIDE THE ISS

For astronauts inside the ISS, even when not touching the sides of the ISS, I don't think they can possibly be in independent orbits around the Earth, as this would be very noticeable.

For a simple example, suppose that two objects start, say, 20 meters apart from each other, at rest relative to the ISS, and positioned so that they are the same distance from the Earth, and also "side by side" relative to the direction the ISS is traveling.

Then - if they are in independent orbits, then these orbits have to intersect. Twice in every orbit. And they start at maximum separation (because they are at rest relative to the ISS and each other). So they would need to hit each other 12.5 minutes later. And then the orbits would criss-cross so they would change places (when the ISS is the other side of the Earth) and then back again.

So, if they were just moving freely in space independently of the ISS, then all the objects in the ISS would continually drift back and forth by tens of meters, if left unattended.

But they don't do that. Astronauts report that for instance, if you go to sleep reading a book, and the book is hovering in front of you, it is still in the same position when you wake up. That surely would be impossible if you are tethered to the ISS and the book is in an independent orbit about the Earth.

I think this must mean that for slow velocities like this, of order of centimeters per second, then the air resistance dampens them so much that the objects stay still. While for larger speeds of orders of meters per second (couple of orders of magnitudes faster) when the astronauts move around in the ISS, then the air resistance is negligible.

Presumably if you had a vacuum inside the ISS, then they would keep moving back and forth like this.

I've tried to find videos of objects moving around in the ISS to see if I can spot them slowing to a stop, if launched at very low velocities with high drag. But can't find a good example yet, and don't seem to be able to turn up  paper on this. Anyone know for sure if this is the explanation of why the astronauts and the objects in the ISS don't seem to be in independent orbit around the Earth?

Any corrections or comments do say, as usual!This originated in my answer to: How practical is it to put a satellite to orbit ISS? on Quora.

If you enjoyed this, you might also like these other posts from my blog: