The new article is titled "An Explicit Embedding of Gravity and the Standard Model in E8". In it, Lisi shows explicitly how the algebra of the gauge fields (the forces of Nature) acting on fermions (matter) can be described by real matrices (arrays of numbers) which relate with elements of the very special group called E8. Why is this relevant for theoretical physics ?
The unification of the mathematical description of the forces of Nature in a single structure is a Holy Grail for theoretical physics, ever since the Standard Model was discovered. The observation that the different strength of the four forces may be an accident of our living in a cold universe brought theoreticians to speculate that we see these forces as distinct, while in reality they are different manifestation of the same "unified field".In the past, attempts at achieving a unified picture of the forces by choosing the "natural" SU(5) group to derive the symmetry groups SU(3) and SU(2)xU(1) that describe respectively quantum chromodynamics and electroweak groups were thwarted by unwanted implications that were in unsolvable conflict with experimental observations. Lisi's attempt with the huge E8 group might well be plagued by similar problems, but the mathematics is sufficiently rich that a solution could be found for any apparent inconsistency.
Rather than trying to make sense of the complex algebra contained in the bulk of the paper, it is interesting to read the conclusions that Lisi draws at the end of his new work:
"If the geometry of our universe is fundamentally that of an E8(-24) principal bundle, this symmetry must be broken, with the frame-Higgs part of the connection attaining a vacuum expectation value, and the structure reducing to that of gravity and the Standard Model. [...] it predicts the existence of many new particles, including W', Z', and X bosons, a rich Higgs sector, and the existence of mirror fermions. The charges of these elementary particles [...] are presented in the Elementary Particle Explorer [...] It is a distinct possibility that some of these new particles may be detected at the Large Hadron Collider."
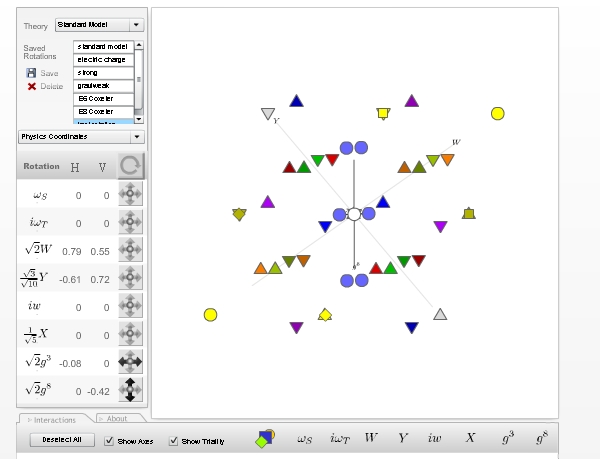The Elementary Particle Explorer! Let's go give a look! Wow. You can play with this tool and make weight diagrams for your theory of choice... Nice, although I do not exactly know what to do with the weights. I guess I have to go back to my notes of Group Theory! Anyway, let us continue with the conclusions (suitably amended):"Although the explicit embedding of gravitiy and the Standard Model in E8(-24) described here is incontrovertible, it raises many questions. Since a direct interpretation implies the existence of mirror fermions, which are not known to exist in nature, it will be necessary to understand how these particles obtain large masses or otherwise work out in the theory. Fortunately, the embedding in E8(-24) also predicts the existence of axions and other Higgs scalars, which interact with the mirrors. These scalars could also help explain the existence of the three generations of fermions - possibly as scalar-fermion composites- and their masses. [...]"
So, it is always the same old story... We have not found supersymmetric particles nor mirror matter, so these things are either hidden from our view (by having a large mass for some strange occurrence), or the theory is wrong. Too bad, but still, a fascinating hypothesis.
He concludes with a note of hope: there is work to do, but it is promising:
"The explicit embedding of gravity and the Standard Model [...] described here provides a solid base from which to develop these ideas further. [...] From the solid foundation of these explicit matrix representations and their embeddings, we might get a better perspective on many of the deep open questions remaining."
Good job. A ray of sunshine in the present landscape, and a boost to the hope to understand much more about Nature within our lifetimes!
Comments