A transverse cut-away view of the CMS detector is shown below, with the different signals that arise from the interaction of different particles. From this view you may well observe why CMS was named "compact muon solenoid": it is the huge external muon detector system (the detectors within the "red" iron slabs on the right) what gives CMS its appearance. Muons have been quite important in the detection of a Higgs boson decay signal, and would have been crucial to discover the Higgs if the elusive particle had a larger mass -in which case the decay to photon pairs would have been invisible, and the decay to two Z bosons would have been the only one granting a full mass reconstruction of Higgs candidates.
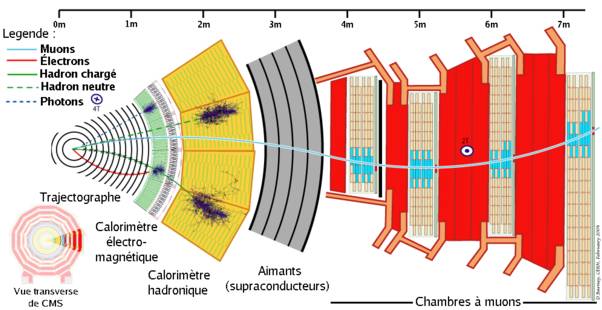
Muons are important, but they are not copiously produced in proton-proton collisions. What comes out of the interaction point is in fact, in most cases, a dijet event. Pairs of jets of light hadrons are the result of two quarks hitting each other hard, and kicking one another out of their parent proton. Quarks cannot live as free objects, and as they exit the proton the strong force pulls them back with an increasing force, causing them to decelerate and popping out of the vacuum additional quark-antiquark pairs. What we observe is a collimated stream of protons, pions, kaons: particles formed by quark-antiquark pairs or by quark triplets. These we call "jets".
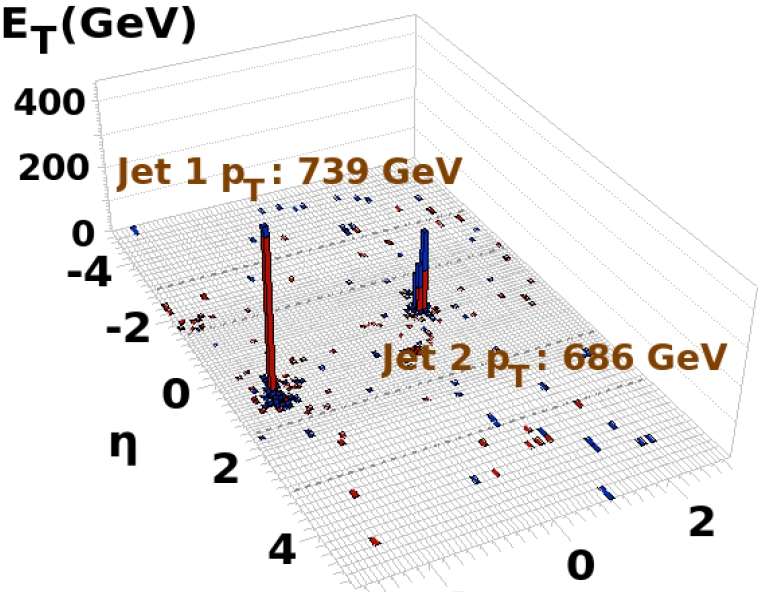
The picture above is a good example of how we like to represent jets as our detector sees them: we imagine the detector is a cylinder, surrounding the interaction point and coaxial with the beam line where protons run. We then cut a side of the cylinder parallel to the beam, and unroll it. We obtain a rectangle, and proceed to plot on it bars which have a height proportional to the amount of energy that the detector measured in each location. This way the streams of particles stand up as towers, well showing that the hard interaction had the main result of producing these two phenomena on opposite sides of the interaction.
The physics of jets is anything but boring; and yet we usually discard most of these events already at trigger level, i.e. during the online data acquisition phase. The reason is that we have had a chance of studying in great detail the mechanism of jet production in past experiments. The precious bandwidth leading to the storing of all information to disk is saved for rarer events featuring particles seen less often in hadron collisions -muons, for instance. CMS can write the full reading of its detector components about 300 times a second, so a draconian selection needs to be enforced, rejecting about 20 million "uninteresting" collisions every second. A sad necessity.
But we do not discard jet events regardless: these in fact remain extremely interesting when their energy is very high ! Past experiments have not had a chance of studying events where the energy available to create new states of matter was higher than about one TeV (a thousand proton masses). With 8 TeV of center-of-mass energy the LHC can extend the reach by about a factor of four with respect to the Tevatron collider. If we have not found any new state of matter produced in quark-antiquark interactions or gluon-gluon collisions with a mass M<1 TeV we still want to see whether such things exist with masses 1<M<4 TeV!
So CMS saved to disk events with high-energy jets, and later filtered them and analyzed them, reconstructing the energy of the two main jets of particles as reconstructed by the various detector elements. Here I am not just talking about the calorimeters: CMS uses a very effective "particle flow" algorithm which reconstructs the energy of every particle making up the jet, using to the fullest the information available from the inner tracker, the electromagnetic and hadronic calorimeters, and the muon chambers.
Once the energy of the two hadronic jets is known, one computes the "dijet invariant mass": this is the mass of a hypothetical particle produced in the collision, if it decayed yielding the two observed jets of hadrons. Of course if there is no particle, but just pairs of quarks kicked out of the collision point by the strong interaction, one does not expect to observe anything else than a smoothly falling histogram of dijet invariant masses. If, however, some events do originate from the production of a new particle, they will produce an extra "bump" in the spectrum.
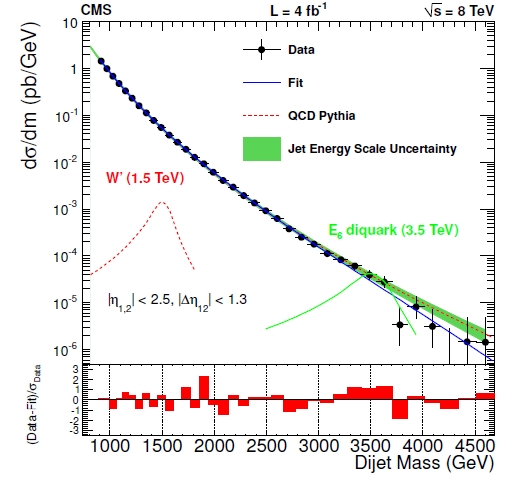
Above, the CMS data (black points) is compared with a Monte Carlo prediction (labeled "QCD Pythia"). Overlaid are two different possible new physics signals: the expected signal from a W' boson (in red, left) and the expected signal of a E6 diquark (in green, right). Both these signals would be detectable in the 4 inverse femtobarns of 8-TeV collisions analyzed by CMS, and are excluded by the search. The lower panel shows the residuals resulting from subtracting from the data the fitted background: there is no significant bump anywhere.
It is the search for such a bump in the spectrum that allows CMS to make a statistical inference on the existence of new particles decaying to hadronic jets. A fit to the observed spectrum can be attempted with a "theory-inspired" functional form which is featureless enough and yet captures the varying slope. By a Bayesian statistical procedure the likelihood of the data, together with a flat prior belief on the intensity of a unknown new particle signal, produces a "posterior distribution" of the possible signal strength. This is always compatible with zero, so there is no evidence of a new particle in the data. For all mass hypotheses of the new particle a 95% confidence level on the signal strength is finally derived by finding the strength below which lies 95% of the area of the posterior distribution.
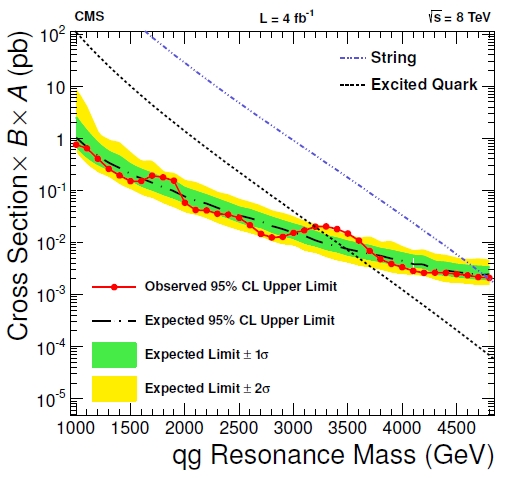 The result is then a set of 95% upper limits on the cross section of the new particle production. This can be represented as a curve in the cross section versus mass graph, as in the figure on the right: all cross sections above the red curve are excluded by the observed data. By comparing the curve with the predicted cross section of new particle production -which is a quickly falling function of the invariant mass of the particle, and is here shown with two different set of dashes for two models- one can then make a statement on the excluded masses: masses smaller than the mass value where the theoretical cross section crosses the 95% upper limit are excluded by the search.
The result is then a set of 95% upper limits on the cross section of the new particle production. This can be represented as a curve in the cross section versus mass graph, as in the figure on the right: all cross sections above the red curve are excluded by the observed data. By comparing the curve with the predicted cross section of new particle production -which is a quickly falling function of the invariant mass of the particle, and is here shown with two different set of dashes for two models- one can then make a statement on the excluded masses: masses smaller than the mass value where the theoretical cross section crosses the 95% upper limit are excluded by the search.Since different hypothetical particles have different predicted production rates, there are several lower limits on the particle masses to derive from the CMS search. We learn that Randall-Sundrum gravitons must have a mass larger than 1.45 TeV if they exist; that a string resonance must have a mass above 4.78 TeV to have escaped detection; and that no Colorons are there with masses below 3.27 TeV; the limit on an excited quark are instead M>3.19 TeV. More detail is of course available in the CMS preprint.
So, all in all we learn that despite having increased the search range by a factor of four since the Tevatron era, the LHC does not see new resonances. There appear to be no fishes waiting to be pulled up in that energy range. For many, it is a disappointment; I am also disappointed in a way, but I at least did not delude myself into thinking we would have caught fishes wherever we cast the net at the LHC!




Comments