Fake Banner
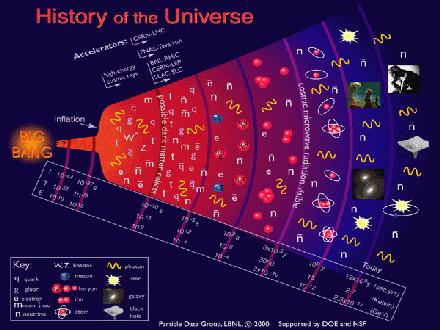
On Progress

The human race has made huge progress in the past few thousand years, gradually improving the living...

The Night Sky From Atacama

For the third time in 9 years I am visiting San Pedro de Atacama, a jewel in the middle of nowhere...

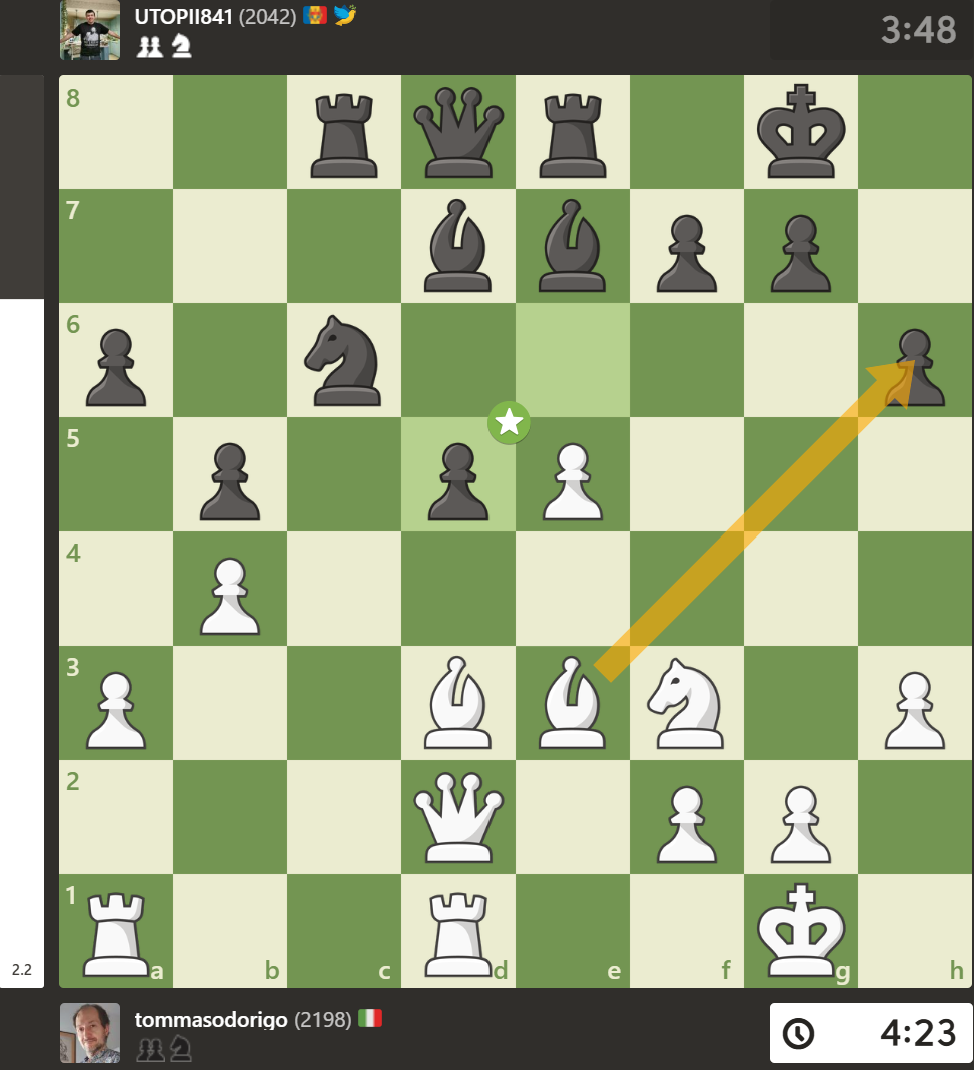On A Roll

What? Another boring chess game?Buzz off, this is my blog, and if I feel like posting a chess game...

When The Attack Plays Itself

After a very intense day at work, I sought some relaxation in online blitz chess today. And the...

User picture.
picture for Hank Campbellpicture for Heidi Hendersonpicture for Bente Lilja Byepicture for Sascha Vongehrpicture for Patrick Lockerbypicture for Johannes Koelman
Tommaso DorigoRSS Feed of this column.

Tommaso Dorigo is an experimental particle physicist, who works for the INFN at the University of Padova, and collaborates with the CMS and the SWGO experiments. He is the president of the Read More »

 Blogroll
Establishing Benchmarks For Use Of AI In Fundamental Science: Two Proposals To Move Forward

Establishing Benchmarks For Use Of AI In Fundamental Science: Two Proposals To Move Forward

Oct 08 2024 | comment(s)

These days I am in the middle of a collaborative effort to write a roadmap for the organization of infrastructures and methods for applications of Artificial Intelligence in fundamental science research. In so doing I wrote a paragraph concerning benchmarks and standards.
Optimization In Valencia

Optimization In Valencia

Sep 28 2024 | comment(s)

Last week I was in Valencia, to attend the fourth MODE Workshop on Differentiable Programming for Experiment Design. It was a great meeting, with 80 participants eager to discuss their latest results in application of complex deep neural network models and similar concoctions to problems in fundamental science. 
Of particular significance is the fact that the average age of the participants was somewhere between 25 and 30 years. In my opening speech I made the point that given the downward trend of that number, soon we will be running a kindergarden. But nobody laughed - these kiddos are serious about machine learning, and they showed it with the excellent quality of the material they presented.
A New Gamma Ray Observatory In Northern Chile

A New Gamma Ray Observatory In Northern Chile

Sep 15 2024 | comment(s)

The SWGO Collaboration (SWGO stands for Southern Wide-Field Gamma Observatory) met this week in Heidelberg, hosted by the Max Planck Institute for Nuclear Physics (MPIK) to discuss progress in the many activities that its members are carrying forward to prepare for the finalization of the design of the observatory and the following construction phase. 
As a member of the collaboration I could learn of many new developments in detail, but I cannot discuss them here as they are work in progress by my colleagues. What I can do here, however, is to describe the observatory as we would like to build it, and a few other things that have been decided and are now public. 
Has Quark-Gluon Plasma Been Observed Yet?

Has Quark-Gluon Plasma Been Observed Yet?

Sep 04 2024 | comment(s)

I will start this brief post with a disclaimer - I am not a nuclear physicist (rather, I am a lesser being, a sub-nuclear physicist). Jokes aside, my understanding and knowledge of the dynamics of high-energy nucleus-nucleus collisions and the phases of matter that can exist at those very high densities and temperatures is overall quite poor. 
Proposal: Call Skoton The Dark Photon

Proposal: Call Skoton The Dark Photon

Aug 19 2024 | comment(s)

I am presently in Cairns, sitting in a parallel session of the "Quark Confinement and the Hadron Spectrum" conference, where I am convening a session on Statistical Methods for Physics Analysis in the XXI Century, giving a talk on the optimization of the SWGO experiment, and playing the piano at a concert for the conference, in addition of course to visiting the area. Anyway, all of the above is too much information to you, as this post is about something else.
Antimatter Over Eurasia

Antimatter Over Eurasia

Aug 08 2024 | comment(s)

Last week I traveled from Venice to Tokyo through Zurich, and during the flights I could do some more tests of the RadiaCode 103 - the nice spectrometer for gamma radiation I have been playing with as of late (for a couple of earlier posts and tests see here and here).