Already a difficulty arises if we want to discuss these properties. What is spin? Spin can be colloquially likened to a measure of how much a body rotates around some axis. However, for elementary particles that is a nonsensical description, as an object which is pointlike, and thus has no structure or features that may allow one to distinguish its orientation, cannot rotate. Around what, if it is pointlike? Still, we have no better way of describing what spin is. So when we draw diagrams to help figuring out the mechanics of particle interactions, we sometimes stick with representing particles as small circles with an arrow crossing them - the arrow representing the direction of the axis around which they are supposed to rotate.
Particles with unit integers of spin - bosons, as mentioned above - are named after an Indian scientist, Satyendra Nath Bose, who first understood the peculiar properties of aggregates of those particles. On the other hand, particles with half-integer spins are called fermions after Enrico Fermi, who likewise understood the quantum properties of that class of particles. The distinction has profound consequences, as fermions are today understood as the elementary constituents of matter, while bosons are the carriers of interactions between them.
In the Standard Model, the elementary particles are either quarks and leptons (fermions), or force carriers (bosons). These are the photon, the W and Z bosons, the gluons, and the Higgs boson. But particles need not be elementary to retain a meaningful classification as fermions and bosons: in fact, the proton - which is by no means elementary, being a composite of three quarks - exhibits a 1/2 spin and is thus a fermion. The same can be said about the neutron. Instead, pions and other particles composed of a quark and an antiquark are bosons, as the two 1/2 spins of their constituents add up to either 1 or 0.
You can understand this rule of addition if you consider standing on a ladder: you can change your height by going up or down by one step at a time. If one step equals a change by 1/2 in your spin, you can e.g. start at zero, take two steps up and one step down, and end up with a 1/2 spin; this is what happens with protons and neutrons. If you instead take one step up and one down, you return where you were before - spin zero; or you can take two steps up, and end up with spin one. The latter two options are available to pions and other particles composed of quark-antiquark combinations.
In LHC particle collisions, the spin of the particles we produce is sometimes important, but it is hardly as significant in determining observable properties of the collision events we observe as it is in what are called "Bose-Einstein correlations". Bose-Einstein correlations arise when we study bosons such as pions: their quantum properties make it more probable to find more such particles with the same quantum attributes, produced in the same collision. This is a quantum correlation: the fact that we see a pion with certain attributes, and e.g. traveling away from the collision in a certain direction, influences the probability to observe another particle nearby, with the same characteristics.
Bose-Einstein correlations have been observed in collisions at a number of facilities in the past, and the LHC also allowed their study since its start in 2010. In fact, one of the very first scientific articles based on real CMS collisions data reported on a measurement of Bose-Einstein correlations, and their characteristics, in events at different collision energies up to 2.36 TeV produced in the very first weeks of running. I was among the main authors of the two publications we produced on that topic. Later, CMS also studied a larger dataset at the higher center-of-mass energy of 13 TeV. So now I am pleased to see that Bose-Einstein correlations are again at the focus of researchers, this time from the competing ATLAS experiment.
The ATLAS paper contains loads of informative graphs, which I hesitate to comment on as they are quite technical in their content. I will only show one graph which shows the correlation effect itself, seen as a enhancement of a correlation function above the expected value of 1.0 (no correlation) at small values of Q - a kinematic variable connected with the difference in momentum of pairs of particles. Small Q means particles with the same kinematics, i.e. ones for which a Bose-Einstein effect is expected.
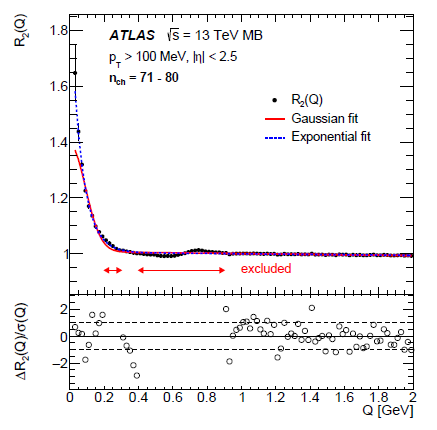
As you see above, the departure from unity of the data (black points in the upper panel, with different models fitting the excess), for small values of Q, is quite large. This enables detailed studies of the effect in a number of ways. By studying the effect, we gain access to important information on the dynamics of the collision. Of course this is not Higgs physics - not as exciting to many of us, at least. But it is still quite important to do our utmost to measure these quantum phenomena wherever we have access to them. So, congratulations to ATLAS for not ignoring this pecular bit of particle physics! If you want to read more about the analysis, it is available on the preprint archive.



Comments