I know that combinations of the searches are already of public domain -for instance, you can read a nice summary in the physics world site. I will also write about that in due time. However, I think it is important to know what are the ingredient used to make a sausage if you want to decide whether you eat it or not. In the case of Higgs boson searches, one has better at least understand what are the analyses that entered the combination. I have limited PC time during my current holidays -see on the right the small angle of paradise I visited this morning, the Preveli beach- so I can only write about CMS results. For ATLAS ones, you can look elsewhere, or forget about it: after all ATLAS produced quite similar results, which turned out to be only a tad less sensitive to the presence of the Higgs.So let me go back to H->ZZ->4l. First of all, what we are searching for is one of the cleanest signatures of Higgs decay, because four energetic, isolated leptons (electrons or muons) are only produced by two other processes in proton-proton collisions according to the Standard Model: pair-production of non-resonant ZZ pairs, or single Z production associated with jets, when jets by unlucky chance produce a signature which mimics a charged lepton.
The search is performed starting from a data sample containing four leptons with very loose kinematic requirements: the range of Higgs masses investigated is from 110 to 600 GeV, and for M(H)<180 GeV one of the two Z bosons emitted in the H->ZZ decay is "off mass-shell", so it produces leptons of moderate or low transverse momentum when it in turn decays. One lepton pair must make an invariant mass loosely compatible with a on-shell Z (60-120 GeV), and the other must only be below 120 GeV.
Two other requirements are crucial to purify the sample: leptons must be isolated from other energy deposits in the calorimeter or charged tracks, and their tracks must precisely point to the primary interaction vertex. The first of these two requirements is aimed at reducing backgrounds from hadronic jets; the second targets leptons produced in the decay of heavy flavour quarks, which would yield leptons with large impact parameter.
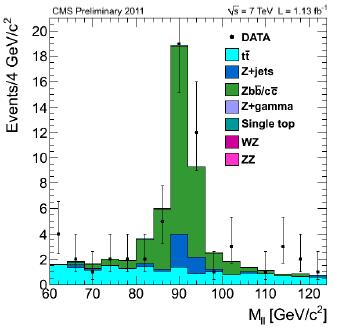Backgrounds are studied with care in control regions which are enriched in each SM contribution. I will just flash here a nice figure which shows how events where two of the four leptons actually originate from bottom-quark decay are flagged and their number compared with simulations for an important cross-check. The distribution on the right shows the invariant mass of the best lepton pair, when two additional leptons have large impact parameter and other isolation requirements loosened up. From the agreement of the data (black points) with the sum of expected backgrounds (the most important being Zbb, in green) one earns confidence in the simulation and estimates a systematic uncertainty on the Monte Carlo-driven background rate.In the end, two different selections are defined: the first is called "baseline", and accepts four-lepton events passing all cuts and with the "looser Z" having an invariant mass above 20 GeV; the other is the "high-mass" selection, where both Z boson candidates must have invariant mass above 60 GeV.
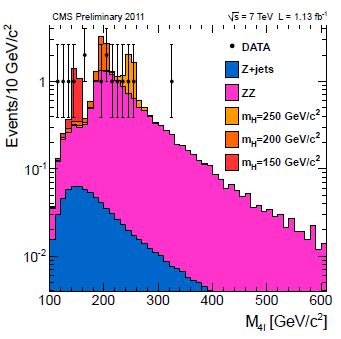You can see the four-body mass of baseline selection in the inset on the left. Many things can be observed here. First of all, you notice that backgrounds shown only belong to the two categories I mentioned earlier: all other SM processes are really irrelevant in this distribution after all cuts (one of the processes that could in principle be present but has been killed effectively is top pair production, where two leptons come from two W boson decays and two additional ones may come from bottom quarks). Second, with three shades of red you see three possible Higgs decay signals shown as they would show up in such a graph. Their normalization corresponds to the expected yield, and their width is the one they would have given the energy resolution on electrons and muons. Another thing to observe is that CMS sees several 4-lepton candidates with low combined mass. In such a figure it is actually rather hard to guesstimate by eye whether there is an excess of events over predicted backgrounds, because the bin size is quite small and the data have mostly empty bins. So let us turn to the computed integral of background events that CMS expects at low mass: six events with mass below 180 GeV are seen, with 1.9 expected. This might appear as interesting news, but the six candidates are scattered in mass, so they cannot be considered a tentative signal.
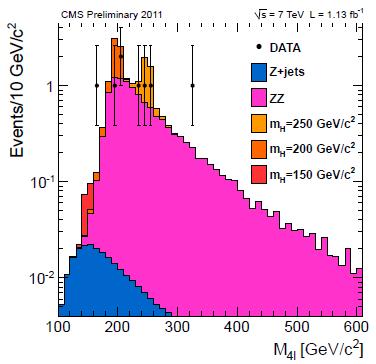On the right you can see the high-mass selection alone: here things are in better agreement over all the spectrum. Maybe the only thing to note here is that CMS has one event with a mass in the whereabouts of 325 GeV, which is where CDF observes four candidates -a fact which has been noticed by a reader here yesterday, and on which my colleague A.Mitra has devoted a slide in a talk summarizing all the recent results by CDF, as shown in the figure below.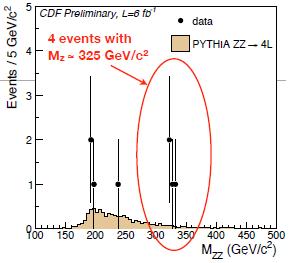
What to say of the four guys at 325 GeV ? I'd say nothing at all -they can't be claimed to be the signal of a new particle, at least until more data confirms it or other experiments see the same thing. So let us move back to the CMS result I am discussing today.
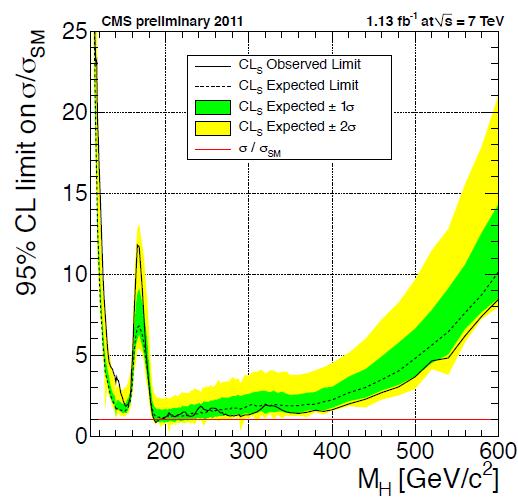From the comparison of event counts in the two 4-lepton selections shown above with expected backgrounds, CMS extracts a limit on the cross section of Higgs production, computed in units of the Standard Model predicted cross section. Such a limit would exclude the existence of the Higgs boson, if it went below 1.0 for any particular mass value. As you see, it does go below 1.0 for a very narrow mass region; overall, the sensitivity to these golden decays of the Higgs is not yet sufficient, given the data collected so far. The result, however, is used in combination with the others I described yesterday, and indeed it allows the exclusion of a large range of Higgs boson masses. I will discuss that tomorrow.
Comments