Special Guest Post From A Far Boundary Of Our Universe

By Richard P. Flatman

"I call our world Flatland, not because we call it so, but to make its nature clearer to you, my happy readers, who are privileged to live in Space."

This is how my great-grandfather, Albert Square, started his memoirs. Memoirs he wrote in solitary confinement. Years later he died, still imprisoned and alone, and unaware that his ideas slowly but steadily started to change the views and imagination, not only of his fellow Flatlanders, but also of you Spacelanders.* 
 
How time has progressed! My great-grandfather is now regarded a science martyr and a hero. A visionary thinker who has shown us Flatlanders the route towards scientific enlightenment. His legacy triggered an avalanche of scientific growth, and an unstoppable progression of insights. 

It is the latest of these insights that I would like to share with you: the remarkable discovery of the strange inner workings of our microscopic world. I, Richard Flatman, have played no minor part in this development. How proud my great-grandfather would have been!


Cover of my great-grandfather's memoirs (6th Spaceland Edition)

Zooming in on Flatland

Our universe is two dimensional. A planar sheet that stretches endlessly. However, when thinking about Flatland, you should not imagine an infinite structureless sheet, but rather an infinite grid of microscopically small cells. These cells have a sixfold symmetry and nicely fit together in a hexagonal pattern.** Elementary particles, aptly called hexons, populate this hexagonal grid. All objects, all matter, our own bodies, everything we Flatlanders see, is build from hexons. So if you understand the interactions and behaviors of hexons, you understand the physical reality of Flatland.

When hexons were discovered to be the elementary building block of matter, it was understood that clumps of matter move due to the hexons that constitute the clump jumping from cell to cell on the hexagonal grid. But how does this hopping dynamics work? How can we predict the movements of hexons? Years ago my investigations started with these questions. Little did I know what surprises I would encounter.


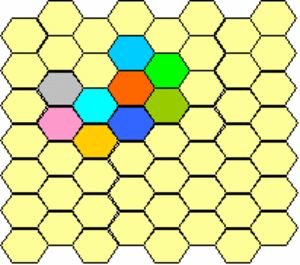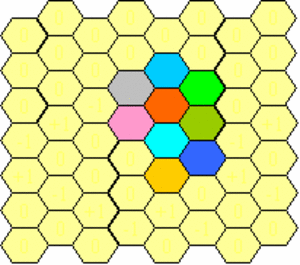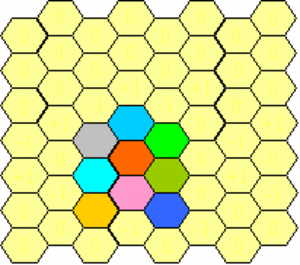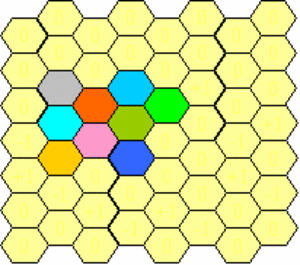
Visualization of a small (eight hexon) lump of matter moving along the Flatland grid

The strange behavior of caged Hexons

I spent years fruitlessly investigating the behavior of hexons. Real progress came when I managed to control planar space at the level of individual cells. This allowed me to isolate and confine single hexons. My first successful experiment is depicted below. I call this set-up a hexon cage, but you Spacelanders might prefer the term hexon billiard. By blocking a few individual cells from the planar grid (the grey cells referred to as scatter centers) I created an area in which a single hexon can rattle around. The figures below depicts what I expected to happen: a hexon trapped forever in a cage formed by the scatter centers. The hexon would follow one of the various specific paths compatible with the shape of the cage. As the dynamics would be too fast to follow, I expected not to see the time-resolved dynamics, but rather the various hexon orbits as depicted.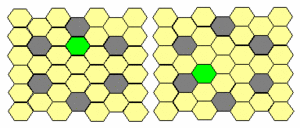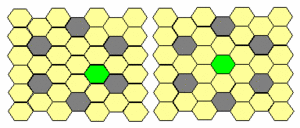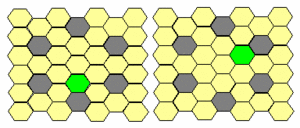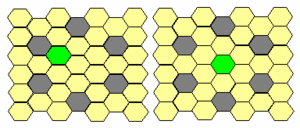


Two expected alternative orbits for a hexon rattling in a hexagonal trap (grey cells depict scatter cells that can not accommodate any hexons)


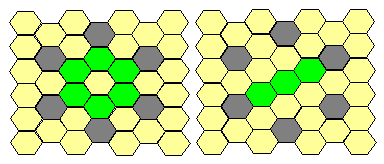
Time average of the above rattling dynamics. For some reason only the hexagonal orbit shown on the left-hand side has ever been observed


To my surprise I observed only one of the these predicted hexon orbits. No matter how hard I tried, I could not get any orbit different from the hexagon loop. This was puzzling. Things became even more puzzling when I realized I had forgotten about other orbits. I had overlooked the simple possibility of a hexon at rest anywhere in its cage. I never observed any such single cell 'orbit'.

Was something wrong with the experiment? To find out, I started building alternative hexon cages. I immediately encountered further surprises. Against expectations, some of the cages built did not result in any trapped particles. Even more puzzling, I hit upon cages incapable of trapping particles, that become capable of doing so by blocking cells in the cage. This really puzzled me. If a container can not hold a certain object, how can the removal of some of the space in the container render it capable of holding that same object?


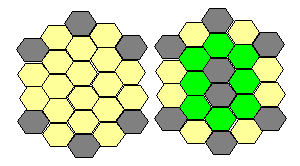
A 'racetrack cage' incapable of holding a hexon (left), and the same cage with some inner cells removed holding a hexon (hexon orbit shown in green)

Other experiments were even more puzzling. While I was sure at any time there was no more than one single hexon in the trap, in some cases I observed clearly separated orbits. What is happening here? Did I witness some form of hexon splitting?***


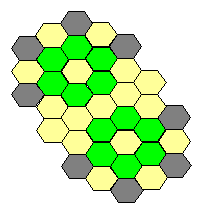
A trap showing hexon orbit splitting. This pattern forms despite only one hexon being trapped.

Shortest paths

As I started contemplating these results, I remembered a recent theory from a fellow Flatlander. The theory was supposed to be an alternative description of the known laws of mechanics. It states that particles going from A to B always select the shortest path possible. It is a remarkable statement, and it seems to work for large scale objects. When applied to my microscopic experiments, the principle seemed capable of describing some of the orbits observed, in particular the orbits that originated in larger cages. Case in point is the trace shown below that could be described as the shortest path that hits all six scatter centers. Similarly, one could describe the hexagonal orbit observed in the first successful trapping experiment as the shortest path that hits all scatter centers forming the cage. Although it was not clear to me how the shortest path rule could be morphed to describe all observed traces including the one consisting of the double loop, I started to entertain the thought that maybe it all depends on looking at these matters from the right perspective.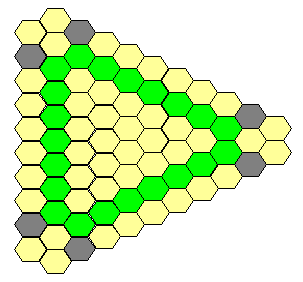 


Hexon orbit observed in a larger cage. The trajectory can be described as the shortest path that hits all scatter cells.
 
Yet I didn't really like the minimum path theory. How would a particle know what lays ahead? And if it doesn't, how can it be able to decide at each step which one would lead to a shorter route? Shortest path selection can only happen if the particle would 'sniff out' the various possible paths. Somehow this idea struck an inner chord, and I tried to work out scenarios for hexons 'sniffing out' their environment.                

Quantum Hexodynamics

What if a hexon tries all possibilities and 'sniffs out' all neighboring cells, and in the next step all neighbors of these neighboring cells, etc? An avalanche of sniffing paths would result. A remarkable thought occurred to me. What if these path to some degree cancel each other, and thereby largely wipe out the avalanche resulting from the sniffing process? 

For cancellation to occur, hexons must have associated with them a number that can be positive as well as negative. Let's assume this number, which I will refer to as the amplitude of the hexon, can take on only two values, +1 and -1. 

So we have a grid of hexagonal cells, and each cell carries an amplitude. This amplitude can be +1 (a cell occupied by an hexon with positive amplitude), it can be -1 (a cell occupied by an hexon with negative amplitude), or it can be 0 (an empty cell). In discrete time steps the hexons jump from cell to cell and thereby carry amplitude across cells. 

Which jumps happen? 

All of them! At each time step each hexon sniffs out all seven possibilities by carrying it's amplitude to each of the six neighboring cells as well as to its own cell. When all hexons have carried their amplitudes in sevenfold fashion, for each cell the resulting amplitude is determined simply by adding all the amplitudes that have arrived at that cell.   

I started drawing hexagonal grids and soon found myself busy experimenting with different amplitude configuration. A cell with a positive amplitude hexon I marked red, a negative amplitude hexon cell blue, and an empty (zero ampIitude) cell faint yellow. As before, the scatter cells used to trap the hexons I colored dark grey. When I started experimenting with different amplitude patterns, the configurations invariably resulted in runaway processes. In all the cases that I tried, the sniffing out led to avalanches that filled the grid with ever larger positive and negative amplitudes. Just as I was about to abandon this pointless 'sniffing idea', I realized that for stable patterns to result, empty (yellow) cells need to be surrounded by an equal number of red and blue cells. This simplified the trial and error process, and almost immediately I stumbled upon a stable pattern. A pattern not hampered by any runaway processes. This pattern is depicted below. Recognize it? Yes, it is the hexagonal trace observed in my first experiment. 


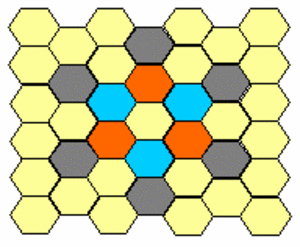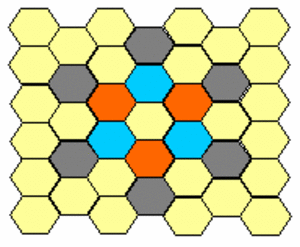
Eureka! A stable path that results when allowing positive and negative amplitude hexons to follow all possible paths. The alternation between red and blue colors represent amplitude sign flipping.

I immediately turned to the racetrack cage, and indeed discovered a stable amplitude pattern is only possible if the center cells contain scatter cells. I then turned to the geometry that resulted in the apparent 'hexon splitting'. I again had to experiment a bit with different hexon amplitude configurations. I soon found a stable configuration: the puzzling two-loop configuration. I knew I had struck gold. The enigmatic hexon dynamics had succumbed. Quantum Hexodynamics (QHD) was born.


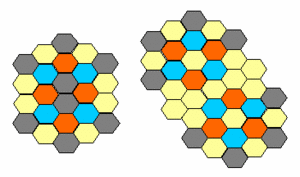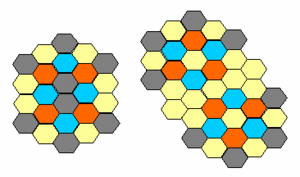
Stable hexon amplitude patterns for racetrack cages.

The simple 'sum over all possibilities algorithm' not only delivers the right hexon paths, it also excludes those paths I initially expected but never observed. A case in point is provided not only by the racetrack cage, but by any of the 'hexon-at-rest' configuration. Each of these lead to a runaway process and don't form a stable configuration.

A Quantum Can Of Worms

It is now several years after the discovery of QHD. It seems the whole Flatland physics community has started working on QHD. Many are working on practical problems and are involved in the computation of stable hexon patterns for geometries of interest. Others are investigating the implications of QHD at a more fundamental level. My friend Werner is mostly intrigued by the sum over all possibilities approach preventing hexons to be localized in one cell.  Key issue is the fact that while the hexon orbits make sense, the occurrence of a hexon at a particular cell is much more enigmatic. QHD predicts that if one attempts to measure the specific location of a hexon, randomness enters the picture. In fact, any cell with +1 or -1 amplitude has equal probability of the hexon showing up, and upon measurement one of these will emerge at random as the cell containing the hexon. I refer to this measurement phenomenon as the 'collapse of the hexon orbit'. Albert, probably the most famous Flatland physicist of all times, abhors this aspect of QHD. He insists that "God doesn't play dice". 
   
Yet, no matter if Albert's God likes it or nor, fact of the matter is: QHD works. Each and every of its predictions that has been put to a test has been confirmed. Yet some aspects of QHD, such as orbit-splitting configurations, are difficult to digest for us Flatlanders. QHD see,s to describe a world for which we can make successful predictions, yet we can not really understand. What is 'understanding' anyway? I prefer to be pragmatic on this issue. I think I can safely say that nobody 'understands' quantum hexodynamics. The advise I give to my fellow flatlanders therefore is: do not keep saying to yourself, if you can possibly avoid it, "But how can it be like that?" because you will get down the drain, into a blind alley from which nobody has yet escaped. Nobody knows how it can be like that.

I just wonder: have you, Spaceland physicists, also landed on a QHD-like theory to describe your microscopic world? And if so, are you bothered about its philosophical implications?  


-Richard P. Flatman-

Notes

* Memoirs published in Spaceland in 1884 under the title "Flatland: A Romance of Many Dimensions".
** It just came to my attention that two Spacelanders recently won a prestigious science prize for nothing more than mimicking our world in a Spaceland lab. Although my initial reaction was one of disappointment (I had assumed your higher dimensional science was well beyond our si ple world), I now realize this opens exiting possibilities for you Spacelanders to create your own versions of Flatland that may even evolve its own life forms!      
*** Despite huge efforts that culminated in the building of the Large Hexon Collider (LHC), no one ever witnessed a hexon splitting up in more fundamental elementary particles.