This year’s Nobel Prize in physics goes to Saul Perlmutter, who shares it with Adam Riess and Brian P. Schmidt, all having been vital in the discovery of that the universe’s expansion is speeding up; research that was done during the 1990s.
Several science bloggers opined that maybe work on quantum entanglement was more deserving, and they have a point, but if the wider impact of scientific issues is at play in the considerations that lead to awarding the Nobel Prize, the decision today was a good one in that regard.

Saul Perlmutter receiving the Shaw Prize for astronomy in 2006 with Brian Schmidt and Adam Riess.
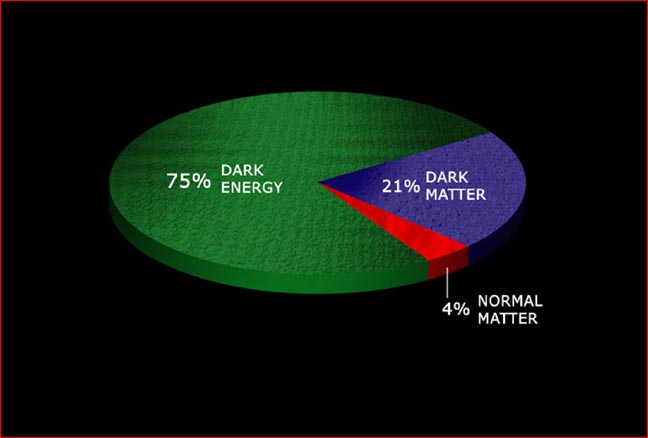
The public and even most physicists (!) totally confuse dark energy with dark matter, the first being an established observational fact; only the second one being a perhaps somewhat dubious assumption (read more about this vital distinction in “Dark Energy, Dark Matter, Dark Force: Not Afraid Of The Dark”).
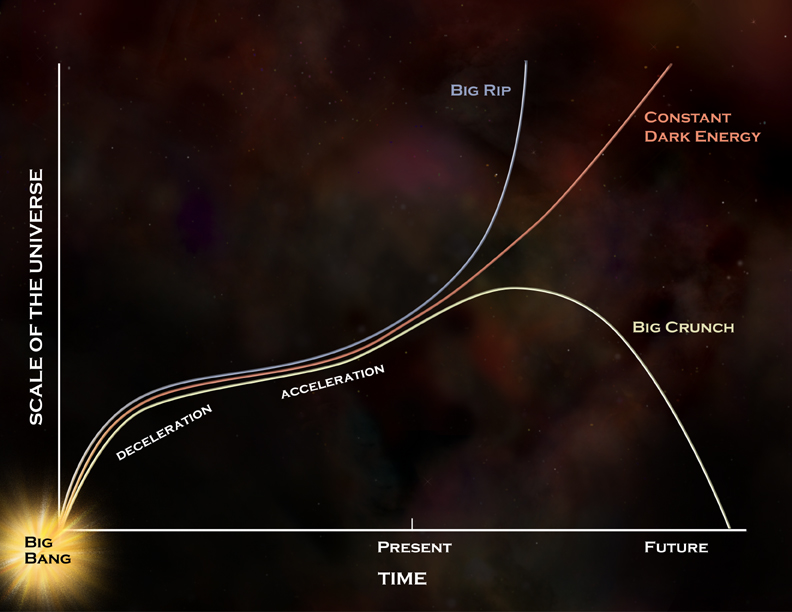
Now with this Nobel Prize, dark energy, aka the cosmological constant*, aka Einstein’s greatest blunder, aka the accelerating expansion of the universe, has gotten its recognition via a Nobel Prize, once more clearly saying for all to take note:
The accelerated expansion of the universe (and thus dark energy) is proper science of the highest quality, period!
Two independent teams - the High-Z Supernova Search Team (headed by Riess) and the Supernova Cosmology Project (Perlmuter) - found that a group of star explosions called Type Ia supernovae were dimmer and therefore farther from Earth than expected.
The light from more than 50 distant stars that went supernova was investigated and turned out far weaker than expected from gravity theory after Einstein tossed the cosmological constant out. Thus, this research touches on another important issue: That one should be very careful with the among lay-philosophers so popular Occam’s (Ockham’s) razor.
Occam’s razor states that given two equally powerful theories, the more parsimonious one with less assumptions should be favored. If aspects are hidden, like for example preferred reference frames in relativity, they could be unnecessary assumptions. Occam’s razor is a popular argument and relates to for example Leibniz equivalence, the parsimony of identifying indiscernible states. Occam’s razor is also one of the most misapplied ideas around, especially considering the ever improving ability to discern previously hidden or ‘unnecessary’ aspects.
 Perlmutter, Riess, and Schmidt being awarded the 2006 Shaw Prize in Astronomy.
Perlmutter, Riess, and Schmidt being awarded the 2006 Shaw Prize in Astronomy.
Occam’s razor must be sparingly applied in order to avoid cutting important aspects, like the cosmological constant (or dark energy term), that further progress may require again and may get you the Nobel Prize! You don’t want to throw a million bucks away, do you?
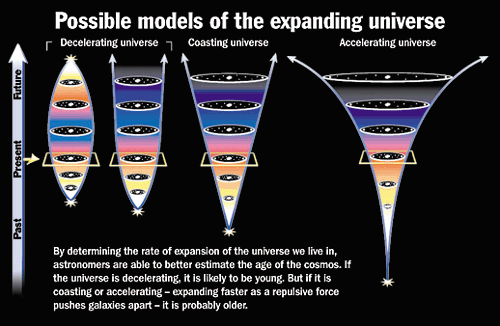
Also important: This discovery was totally unexpected and the researchers thought for a long time that the finding must be a mistake. They were basically just looking into what went wrong (pretty much like the guys with the faster than light neutrinos are doing as we speak, thinking that the data must be a systematic error).
Perlmutter, Schmidt and Riess were trying for a long while to make the accelerated expansion disappear, but the more they analyzed the data, the surer they became that indeed, the universe will expand ever faster, taking all galaxies away from each other, leaving a totally dark and cold sky.
So, in conclusion, I am happy about this year’s choice, because people being afraid of the dark is a little pet peeve of mine. If you would like to know more about why the accelerated expansion of the universe and dark energy are not “modern science losing it and going nuts” but instead down to earth physics that just gotten somewhat of a bad name (literally), I tried my best to explain it also here:
· Not Afraid Of The Dark: Dark Energy As The Ultimate Sisyphus
---------------------------* Remark: Einsteins cosmological constant was constant of course and thought to just hold the universe stable while the dark energy term may conceivably depend on time and is also larger, so that the universe is not just stable against its own gravity, but actually "blows up". Nevertheless, these are all the same term inside the Einstein equations. The observed accelerated expansion is simply telling us how large that term actually is and perhaps even how it depends on time (with better observations).




Comments